Problem C3.2. Turbulent Flow over the DPW III Wing Alone Case

Overview

This problem is aimed at testing high-order methods for a three-dimensional wing case with turbulent boundary layers at transonic conditions. This problem has been investigated previously with low order methods as part of the AIAA drag prediction workshop,

http://aaac.larc.nasa.gov/tsab/cfdlarc/aiaa-dpw/Workshop3/

(see DPW-W1). The target quantity of interest is the drag coefficient at one free-stream condition, as described below.

Governing Equations

The governing equation is the 3D Reynolds-averaged Navier-Stokes equations with a constant ratio of specific heats of 1.4 and Prandtl number of 0.71. The dynamic viscosity is also a constant. The choice of turbulence model is left up to the participants; recommended suggestions are 1) the Spalart Allmaras model, and 2) the Wilcox k-omega model.

Flow Conditions

Mach number M_∞=0.76, angle of attack α=0.5^o, Reynolds number (based on the reference chord) Re_cref = 5x10⁶. The boundary layer is assumed fully turbulent and no wind tunnel effects are to be modeled.

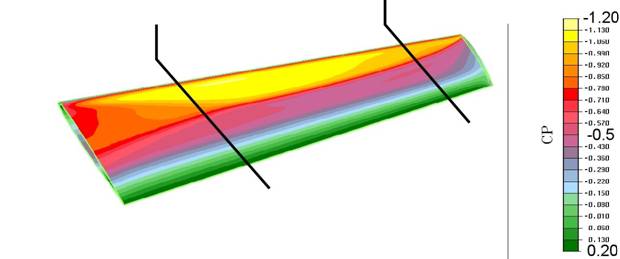
Geometry

The wing geometry, illustrated below with pressure contours, is available online at

http://aaac.larc.nasa.gov/tsab/cfdlarc/aiaa-dpw/Workshop3/DPW3-geom.html

The reference quantities are as follows:

Planform area: S_ref = 290322 mm² = 450 in²

Chord: c_ref = 197.556 mm = 7.778 in

Span: b = 1524 mm = 60 in

Boundary Conditions

Adiabatic no-slip wall on the wing, symmetry at the wing root, and free-stream at the farfield.

Grids

Participants may use their own grids for the convergence study. The initial coarse mesh should yield similar geometry resolution to the coarse meshes provided by the DPW workshop:

http://aaac.larc.nasa.gov/tsab/cfdlarc/aiaa-dpw/Workshop3/grids.html

The grids provided by the workshop, as well as the gridding guidelines,

http://aaac.larc.nasa.gov/tsab/cfdlarc/aiaa-dpw/Workshop3/gridding_guidelines.html

are understood to be relevant to second-order methods. Grids for higher-order methods will likely be coarser for the same level of solution accuracy. However, the geometry must still be represented accurately. For example if curved elements are used, the maximum error in the geometry representation should be similar to the error in the finer linear meshes. For structured meshes, one technique for achieving this resolution requirement is to agglomerate linear elements from the low-order meshes into higher-order, curved, macro-elements. For example a 3x3 block of linear elements can be combined into one cubic curved element, yielding 27 times fewer elements at a similar geometry resolution.

Requirements

1. Perform a convergence study of drag coefficient, c_d, using one or more of the following three techniques:

a. Uniform mesh refinement of the coarsest mesh

b. Quasi-uniform refinement of the coarsest mesh, in which the meshes are not necessarily nested but in which the relative grid density throughout the domain is constant.

c. Adaptive refinement using an error indicator (e.g. output-based).

Record the degrees of freedom and the work units for each data point, where the CPU t=0 corresponds to initialization with free-stream conditions on the coarsest mesh.

2. Submit two sets of data to the workshop contact for this case

a. c_d error versus work units

b. c_d error versus degrees of freedom

Include a description of the coarsest mesh resolution and of the strategy used for refinement.